D. G. Marangon1, G. Vallone1, P. Villoresi1
Phys. Rev. Lett. 118, 060503 (2017)
DOI:10.1103/PhysRevLett.118.060503
1Department of Information Engineering, University of Padova, via Gradenigo 6/B, 35131 Padova, Italy
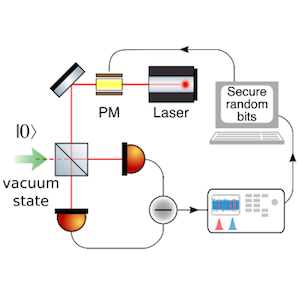
Measurements of the electromagnetic vacuum fluctuations allowed the generation of random numbers at a gigabits per second rate and with an enhanced security level with respect to the state-of-the-art quantum random number generators (QRNG). Random numbers, employed in any cryptographic algorithm, are of paramount importance for security applications. It is well known that physical systems and processes governed by quantum mechanics feature intrinsic non-deterministic behaviours that can be used to generate unpredictable random numbers. With respect to the QRNG state-of-the-art, in this work we designed and realized a novel protocol able to merge an enhanced level of security with an ultrafast rate of generation. The protocol is resilient against an extreme class of “guessing attacks”. Such attacks indeed contemplate the worst case scenario, in which an adversary is able to control the generator quantum source itself, the so-called semi-device-independent (SDI) framework. In our protocol, the Heisenberg Uncertainty Principle is used not only to generate the random numbers, as most of the previous QRNGs do, but also to secure them by erasing any guessing advantage an extremely resourceful adversary might achieve. By applying the SDI approach to the measurement of the vacuum fluctuations of the electromagnetic field, we give an experimental demonstration of secure random number generation at a rate of gigabits per second. Such a result is obtained by employing commercially available hardware and could be easily improved to reach rates of tens of gigabits per second. This novel scheme is able to provide unpredictability at the highest levels, while keeping low the setup complexity and achieving a generation rate able to satisfy the even growing demand of ultrafast streams of secure random numbers.
