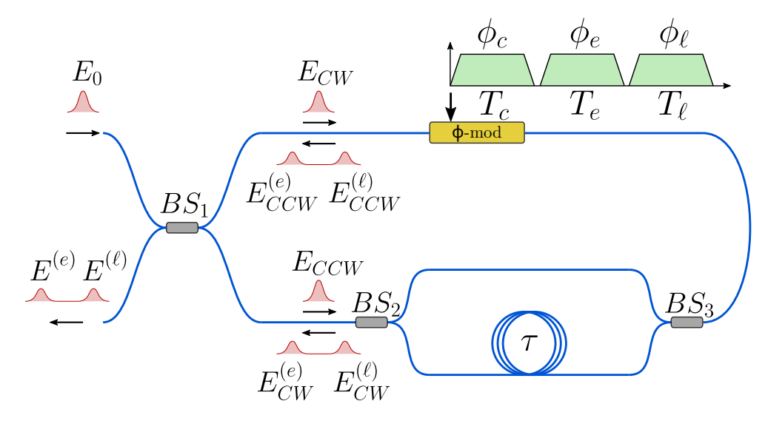
Time-bin encoding has been widely used for implementing quantum key distribution (QKD) on optical fiber channels due to its robustness with respect to drifts introduced by the optical fiber. However, due to the use of interferometric structures, achieving stable and low intrinsic Quantum Bit Error rate (QBER) in time-bin systems can be challenging. A key device for decoy-state prepare & measure QKD is represented by the state encoder, that must generate low-error and stable states with different values of mean photon number. Here we propose the MacZac (Mach-Zehder-Sagnac), a time-bin encoder with ultra-low intrinsic QBER (<2e-5) and high stability. The device is based on nested Sagnac and Mach-Zehnder interferometers and uses a single phase modulator for both decoy and state preparation, greatly simplifying the optical setup. The encoder does not require any active compensation or feedback system, and it can be scaled for the generation of states with arbitrary dimension. We experimentally realized and tested the device performances as a stand-alone component and in a complete QKD experiments. Thanks to the capacity to combine extremely low QBER, high stability and experimental simplicity, the proposed device can be used as a key building block for future high-performance, low-cost QKD systems.
Quantum correlations between the measurements of two or more separated observers play a fundamental role in many applications, such as randomness generation or key distribution. Recently, it was realized that sequential measurements (i.e., defined with a precise temporal ordering between subsequent measurements on a given system) can enhance the performance of these protocols. However, the theoretical understanding of how to maximize this performance is limited and the relation with the boundary of quantum correlations is unexplored. In the case of one party on one side and two sequential parties on the other, we study the geometry of quantum correlations and its implications for robust device-independent randomness generation. We identify a boundary for the set of these correlations expressed as a trade-off between the amount of nonlocality between different observers and show that this allows to generate the maximum possible device-independent randomness in our setting, namely two bits. We propose a practical protocol based on non-projective measurements that can produce the boundary correlations under ideal conditions, and address its robustness to noise, showing that it is improved compared to previous approaches. Finally, we implement our protocol in a proof-of-concept experiment based on a photonic implementation. With the obtained correlations we could certify more bits per state with respect to the standard CHSH protocol, proving that our protocol is feasible and robust to real-world imperfections. Our work paves the way for a full understanding of sequential quantum correlations and their exploitation for practical and efficient device-independent protocols.
